Types Of Coloring In Graph Theory, Exact Coloring Wikipedia
Types of coloring in graph theory Indeed lately is being sought by users around us, perhaps one of you. People are now accustomed to using the internet in gadgets to see image and video information for inspiration, and according to the name of this article I will talk about about Types Of Coloring In Graph Theory.
- Https Www Ripublication Com Ijcam17 Ijcamv12n2 26 Pdf
- How To Find Chromatic Number Graph Coloring Algorithm Gate Vidyalay
- Pdf Colorful Paths In Vertex Coloring Of Graphs
- Https Encrypted Tbn0 Gstatic Com Images Q Tbn 3aand9gcst4tnxqzqelgxfkkpjxtztydslcnyrkv1bxuljmfbd1r8ccj5k Usqp Cau
- Graph From Wolfram Mathworld
- Inventions Free Full Text Graph Theory A Comprehensive Survey About Graph Theory Applications In Computer Science And Social Networks Html
Find, Read, And Discover Types Of Coloring In Graph Theory, Such Us:
- Graph Theory Ch 5 Coloring Of Graphs 1 Chapter 5 Coloring Of Graphs Ppt Download
- Overview Of Graph Colouring Algorithms
- Vertex Coloring Of Graphs Via Phase Dynamics Of Coupled Oscillatory Networks Scientific Reports
- Http Www Ijstr Org Final Print Jan2020 Integrating A Graph Theory In A School Math Curriculum Of Indonesia Under Realistic Mathematics Education Pdf
- Graph Theory And Its Applications
If you re searching for Hello Kitty Cartoon Coloring Pages you've come to the perfect place. We ve got 104 graphics about hello kitty cartoon coloring pages adding pictures, pictures, photos, backgrounds, and more. In such page, we also provide number of graphics available. Such as png, jpg, animated gifs, pic art, symbol, black and white, transparent, etc.
Graph Coloring Wikipedia The Free Encyclopedia Theoretical Computer Science Discrete Mathematics Hello Kitty Cartoon Coloring Pages
Cycle Graph Theory Wikipedia Hello Kitty Cartoon Coloring Pages
Check Whether A Given Graph Is Bipartite Or Not Geeksforgeeks Hello Kitty Cartoon Coloring Pages
Graph Theory Coloring Tutorialspoint Hello Kitty Cartoon Coloring Pages
Four Color Theorem Wikipedia Hello Kitty Cartoon Coloring Pages
Pdf Fall Colorings Of Graphs Hello Kitty Cartoon Coloring Pages
A k edge coloring of g is an assignment of k colors to the edges of g in such a way that any two edges meeting at a common vertex are assigned different colors.
Hello kitty cartoon coloring pages. In the above graph there is only one vertex v without any edge. In a graph no two adjacent vertices adjacent edges or adjacent regions are colored with minimum number of colors. A simple graph which has n vertices the degree of every vertex is at most n 1.
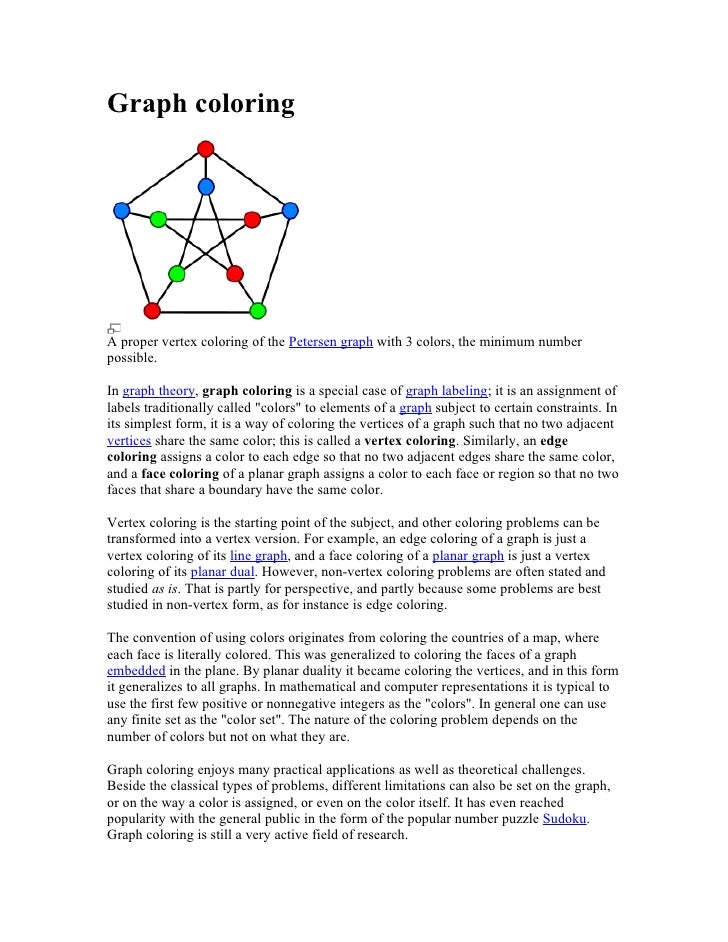
This number is called the chromatic number and the graph is called a properly colored graph. A trivial graph is the graph which has only one vertex. Therefore it is a trivial graph.
We will discuss only a certain few important types of graphs in this chapter. A simple graph is the undirected graph with no parallel edges and no loops. One may also consider coloring edges possibly so that no two coincident edges are the same color or other variations.
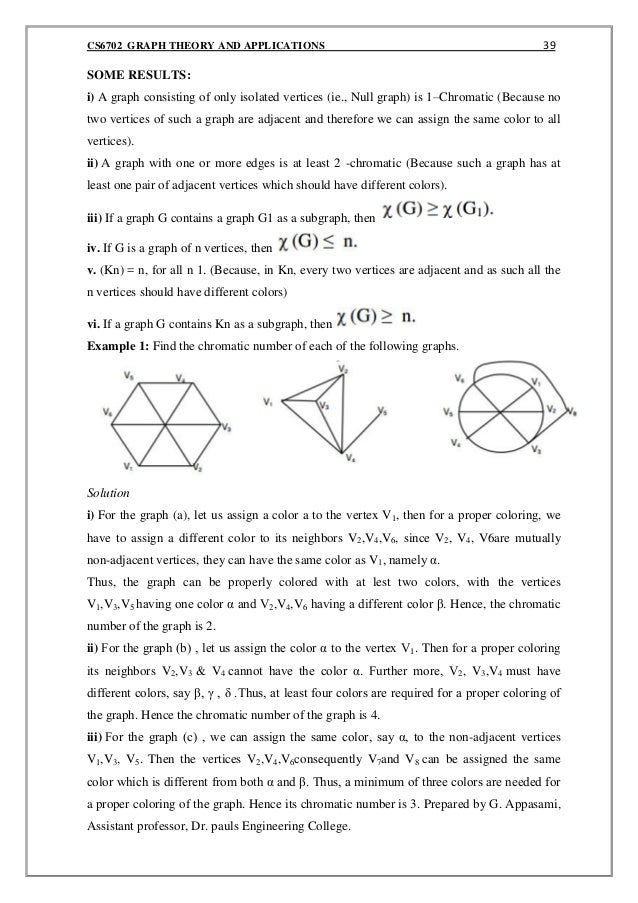
Let g be a graph with no loops. If g is the complete graph k n then p g k kk 1k 2. The smallest number of colors needed for an edge coloring of a graph g is the chromatic index or edge chromatic number x g.
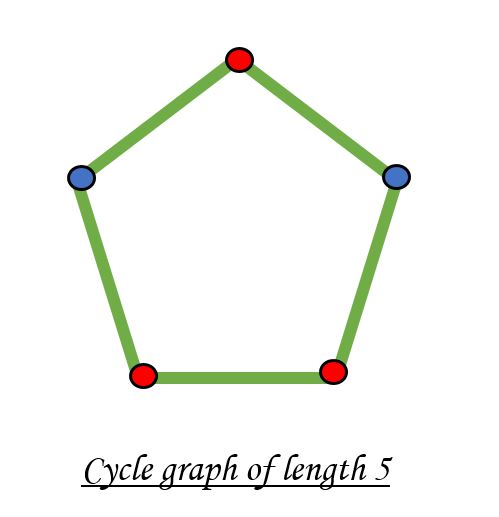
Many problems and theorems in graph theory have to do with various ways of coloring graphs. There are various types of graphs depending upon the number of vertices number of edges interconnectivity and their overall structure. In the above graph there are three vertices named a b and c.
Typically one is interested in coloring a graph so that no two adjacent vertices have the same color or with other similar restrictions. A tait coloring is a 3 edge coloring of a cubic graph. The four color theorem is equivalent to the assertion that every planar cubic bridgeless graph admits a tait coloring.
Math For Seven Year Olds Graph Coloring Chromatic Numbers And Eulerian Paths And Circuits Joel David Hamkins Hello Kitty Cartoon Coloring Pages
Coloring Hello Kitty Cartoon Coloring Pages
Brooks Theorem Wikipedia Hello Kitty Cartoon Coloring Pages
Graph Coloring Hello Kitty Cartoon Coloring Pages
More From Hello Kitty Cartoon Coloring Pages
- Rainy Day Coloring Pages Free Printable
- Free Dinosaur Coloring Pages To Print
- My Little Pony Coloring Book
- Dinosaur Coloring Pages Dltk
- Is Plan G Better Than Plan F
Incoming Search Terms:
- Check Whether A Given Graph Is Bipartite Or Not Geeksforgeeks Is Plan G Better Than Plan F,
- Chromatic Number From Wolfram Mathworld Is Plan G Better Than Plan F,
- Graph Edge Coloring By Tutorcircle Team Issuu Is Plan G Better Than Plan F,
- Chromatic Number Definition Examples Video Lesson Transcript Study Com Is Plan G Better Than Plan F,
- Brooks Theorem Wikipedia Is Plan G Better Than Plan F,
- Overview Of Graph Colouring Algorithms Is Plan G Better Than Plan F,
