Graph Theory Coloring Book, A Short Introduction To Graphs Graphia

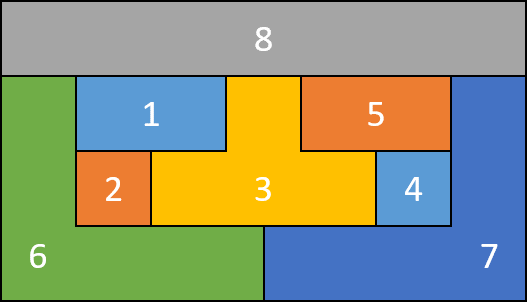
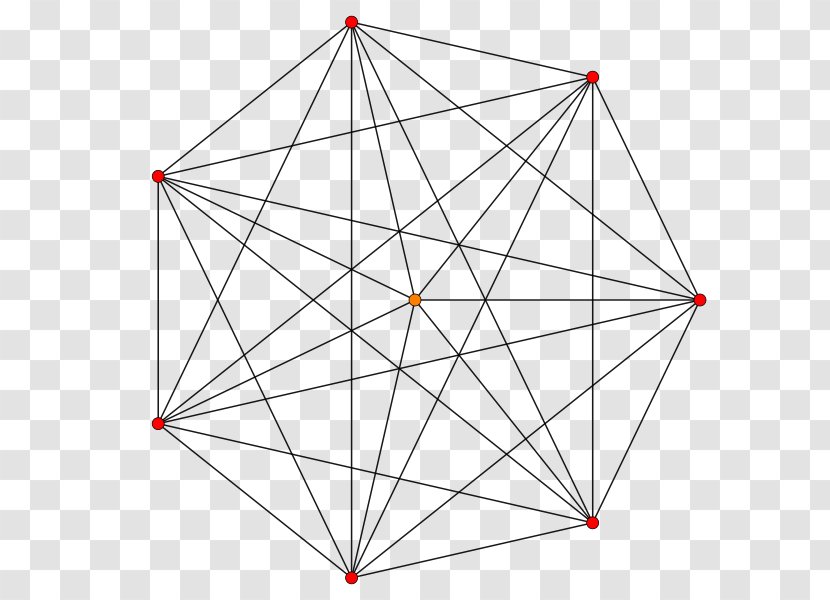
In the complete graph each vertex is adjacent to remaining n1 vertices.

Monkey emoji coloring pages. An introduction to combinatorics and graph theory. Most of the graph coloring algorithms in practice are based on this approach. And almost you could almost say is a generic approach.

The intuitive statement of the four color theorem given any separation of a plane into contiguous regions the regions can be colored using at most four colors so that no two adjacent regions have the same color needs. Hence the chromatic number of kn n. For example you could color every vertex with a different color.

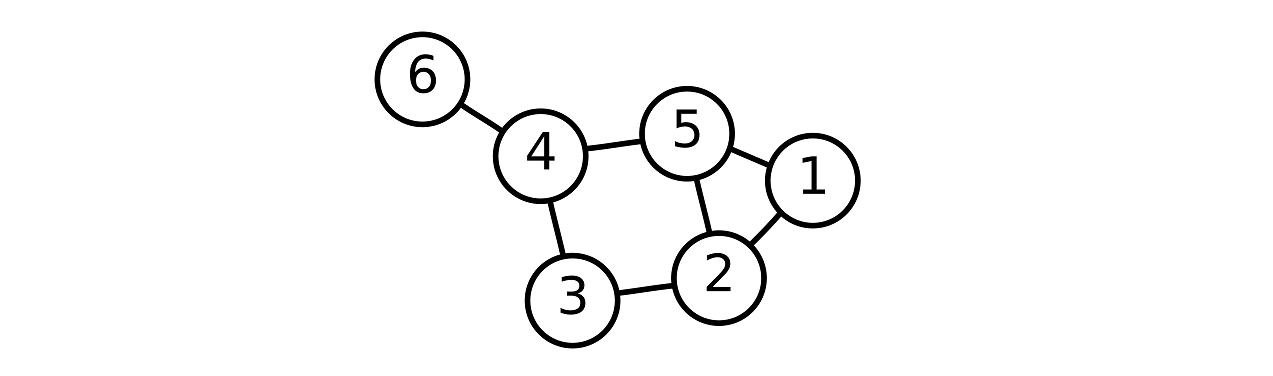
Precise formulation of the theorem. In a graph no two adjacent vertices adjacent edges or adjacent regions are colored with minimum number of colors. This is called a vertex coloring.

So lets define that and then see prove some facts about it. Similarly an edge coloring assigns a color to each edge so that no two adjacent edges are of the same color and a face coloring of a planar graph assign. And that is probably the most basic graph coloring approach.

It is an assignment of labels traditionally called colors to elements of a graph subject to certain constraints. And for our graph g with. Hence each vertex requires a new color.

A k coloring of g is an assignment of k colors to the vertices of g in such a way that adjacent vertices are assigned different colors. Every graph has a proper vertex coloring. Trudeau has created a whole path in this book including planar graphs platonic graphs the genus of a graph hamilton walks eulers formula coloring euler walks and the seven bridges of konigsberg.

If the vertex coloring has the property that adjacent vertices are colored differently then the coloring is called proper. Along with well chosen topics suitable exposition giving a universal touch. The author richard j.

Applications of graph coloring graph coloring is one of the most important concepts in graph theory. Subsection coloring edges the chromatic number of a graph tells us about coloring vertices but we could also ask about coloring edges. Just like with vertex coloring we might insist that edges that are adjacent must be colored differently.

In its simplest form it is a way of coloring the vertices of a graph such that no two adjacent vertices are of the same color. Contains exercises mentioned at the end of every chapter. The adventurous reader is encouraged to find a book on graph theory for suggestions on how to prove the theorem.

This book explains the following topics. Let g be a graph with no loops. If g has a k coloring then g is said to be k coloring then g is said to be k colorablethe chromatic number of g denoted by xg is the smallest number k for which is k colorable.

In graph theory graph coloring is a special case of graph labeling. In general given any graph gtext a coloring of the vertices is called not surprisingly a vertex coloring. Inclusion exclusion generating functions systems of distinct representatives graph theory euler circuits and walks hamilton cycles and paths bipartite graph optimal spanning trees graph coloring polyaredfield counting.

This number is called the chromatic number and the graph is called a properly colored graph. Graph coloring is nothing but a simple way of labelling graph components such as vertices edges and regions under some constraints.