Vertex Coloring Number, Math For Seven Year Olds Graph Coloring Chromatic Numbers And Eulerian Paths And Circuits Joel David Hamkins
Vertex coloring number Indeed lately is being hunted by users around us, perhaps one of you. People now are accustomed to using the internet in gadgets to see image and video data for inspiration, and according to the name of this article I will talk about about Vertex Coloring Number.
- Graph Theory
- Understanding Graph Coloring Vertex A Greedy Approach Part I Thiago Ribeiro
- Print All K Colorable Configurations Of The Graph Vertex Coloring Of Graph Techie Delight
- Https Encrypted Tbn0 Gstatic Com Images Q Tbn 3aand9gctdraly4yfbnndrswq5gwxtr3kax8rkjjpbvu20plhgd0csge4y Usqp Cau
- Math For Seven Year Olds Graph Coloring Chromatic Numbers And Eulerian Paths And Circuits Joel David Hamkins
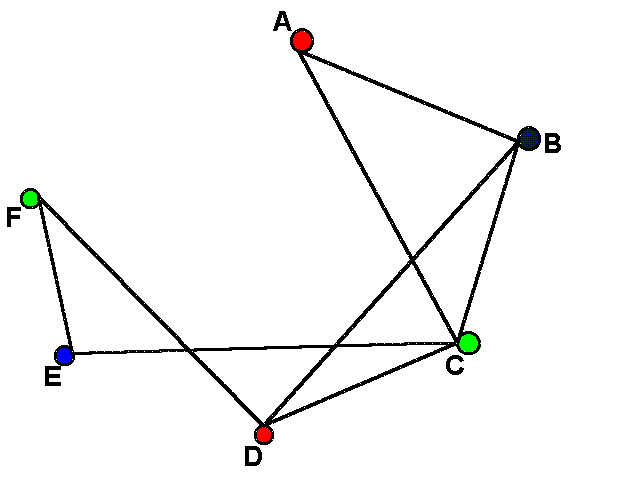
- An Example Of Vertex Coloring Using A Minimum Of 3 Colors And Edges Download Scientific Diagram
Find, Read, And Discover Vertex Coloring Number, Such Us:
- Vertex Coloring Martin Thoma
- Vertex Color Tool For Maya Free Texturing Scripts Plugins Downloads For Maya
- Graph Coloring
- Graph Coloring Set 2 Greedy Algorithm Geeksforgeeks
- Graph Coloring Using Recursive Large First Rlf Algorithm Codeproject
If you are looking for Valentine Religious Coloring Pages you've reached the perfect location. We have 104 images about valentine religious coloring pages adding pictures, photos, pictures, wallpapers, and more. In such web page, we also have number of graphics available. Such as png, jpg, animated gifs, pic art, symbol, blackandwhite, transparent, etc.
This site is related to the classical vertex coloring problem in graph theory.
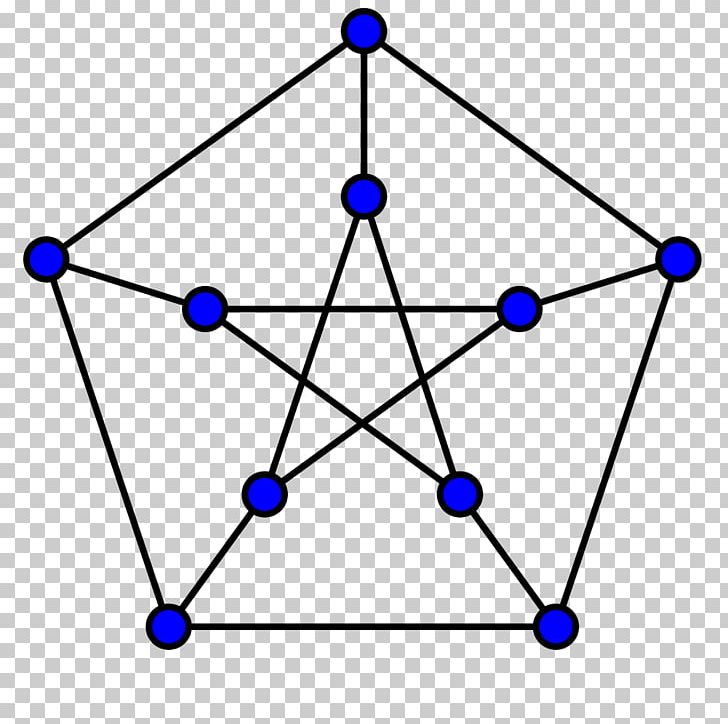
Valentine religious coloring pages. Zero knowledge protocol vertex coloring is relevant for so called zero knowledge protocols. Simply put no two vertices of an edge should be of the same color. Every graph has a proper vertex coloring.
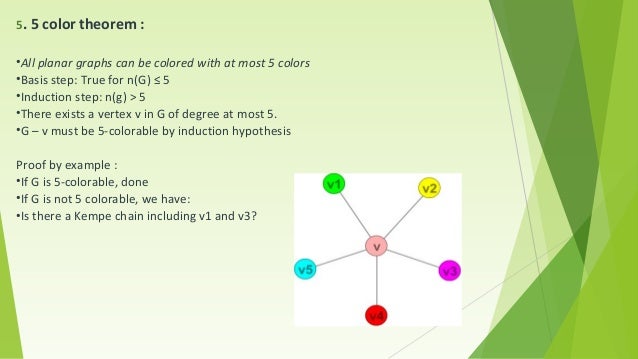
This is what we recognize as vertex colouring. If the vertex coloring has the property that adjacent vertices are colored differently then the coloring is called proper. The minimum number of colors required for vertex coloring of graph g is called as the chromatic number.
See the wikipedia article graphcoloring for further details on graph coloring. Used to count the possible number of ways of properly vertex coloring a graph. But often you can do better.
Vertex colouring and chromatic numbers. Imagine that we could take the vertices of a graph and colour or label them such that the vertices of any edge are coloured or labelled differently. Brelazs heuristic algorithm can be used to find a good but not necessarily.
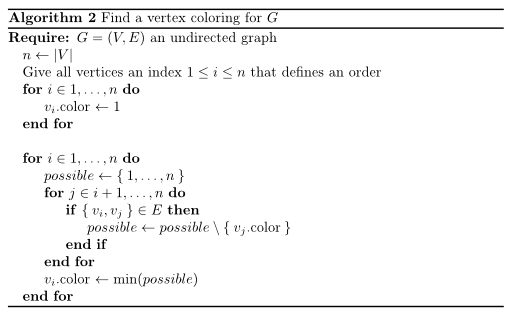
Vertex coloring is an assignment of colors to the vertices of a graph g such that no two adjacent vertices have the same color. We present a new polynomial time algorithm for finding proper m colorings of the vertices of a graphwe prove that every graph with nvertices and maximum vertex degree i must have chromatic number ig less than or equal to i1 and that the algorithm will always find a proper m coloring of the vertices of gwith mless than or equal to i1. Graph coloring benchmarks instances and software.
As soon as you dont get valid colorings you know that the number of colors youve used in the last valid coloring was the minimum number. This function can compute the chromatic number of the given graph or test its k colorability. The minimum number of colors itself is called the chromatic number denoted and a graph with chromatic number is said to be a k chromatic graph.
P3 can be colored in 12 ways using 3 colors. The smallest number of colors needed to get a proper vertex coloring is called the. An edge coloring of a graph is a proper coloring of the edges meaning an assignment of colors to edges so that no vertex is incident to two edges of the same coloran edge coloring with k colors is called a k edge coloring and is equivalent to the problem of partitioning the edge set into k matchingsthe smallest number of colors needed for an edge coloring of a graph g is the chromatic index.
It presents a number of instances with best known lower bounds and upper bounds. For the same graphs are given also the best known bounds on the clique number. For example you could color every vertex with a different color.

Graph Coloring In Graph Theory Chromatic Number Of Graphs Gate Vidyalay Valentine Religious Coloring Pages
More From Valentine Religious Coloring Pages
- Preschool Coloring Crafts
- Palette Coloring Tool
- Valentine Coloring Zone
- Thomas The Train Coloring Page
- Wheel Of Dharma Coloring Pages
Incoming Search Terms:
- Circular Coloring Wikipedia Wheel Of Dharma Coloring Pages,
- Kenneth Rosen Edition 7th Exercise 10 8 Question 23 Page No 734 Gate Overflow Wheel Of Dharma Coloring Pages,
- Vertex Coloring From Wolfram Mathworld Wheel Of Dharma Coloring Pages,
- Vertex Coloring And Chromatic Number Youtube Wheel Of Dharma Coloring Pages,
- Graph Coloring Set 1 Introduction And Applications Geeksforgeeks Wheel Of Dharma Coloring Pages,
- Graph Coloring And Its Applications Wheel Of Dharma Coloring Pages,