Rainbow Coloring In Graph Theory, 2

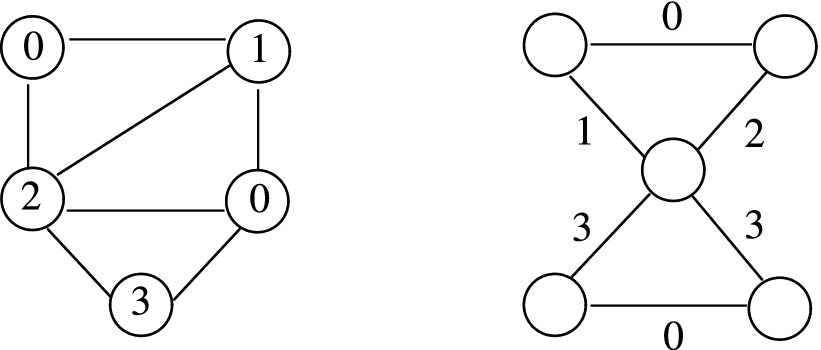
An edge coloring of a graph is a function from its edge set to the set of natural numbers.

Poop emoji coloring. A non rainbow coloring of a plane graph g is a vertex coloring such that each face of g is incident with at least two vertices with the same color. Consequently if g is a connected graph of order n 3 then rmg n. In graph theory rainbow coloring of graphs is an edge coloring technique of the graphs.

In this paper a rainbow coloring of the corona of p n ok 2 the corona of p no c 4 flower graphs and fan graph are considered and rcg of these graphs are decided. In this case we say that the edge e has color s. A total colored graph g is rainbow total connected if any two vertices of g are connected by a path whose edges and internal vertices have distinct colors.

An edge cut r of g is called a rainbow cut if no two edges in r are colored the same. Let g be a nontrivial connected edge colored graph. Rainbow cycle edge coloring hamilton cycle.

A path in an edge colored graph with no two edges sharing the same color is called a rainbow path. C is a rainbow mean coloring of gg. Rainbow mean index rmg of the graph g itself is dened as rmg minfrmc.

Colors required to edge color the graph such that the graph is rainbow connected. Graph colorings is one of the most important concepts in graph theory. Ccc 0364 902493050607 06 608 journal of graph theory in this case rainbow colorings are automatically proper edge colorings in the usual sense ie each color class is the union of disjoint edges.

A concept of rainbow has been used in various fashions in a graph theory context in 123891014151617181920 21 and related papers. A graph is said to be rainbow connected or rainbow colored if there is a rainbow path between each pair of its verticesif there is a rainbow shortest path between each pair of vertices the graph is said to be strongly rainbow connected or strongly rainbow colored. The maximum number of colors that can be used in a non rainbow coloring of a plane graph g is denoted by.

Journal of graph theory vol. In graph theory a path in an edge colored graph is said to be rainbow if no color repeats on it. An edge coloring of g is a rainbow disconnection coloring.

5 607 612 1993 0 1993 john wiley sons inc. In this paper we prove that rtcg can be bounded by a constant 7 if the following. Unlike in the case of ordinary colorings the goal is to maximize the number of used colors.

In the present paper we study the existence of a hamilton cycle with many colors also the existence of a hamilton cycle with few colors in any proper edge coloring key words.