Graph Theory Graph Coloring, Star Coloring Wikipedia

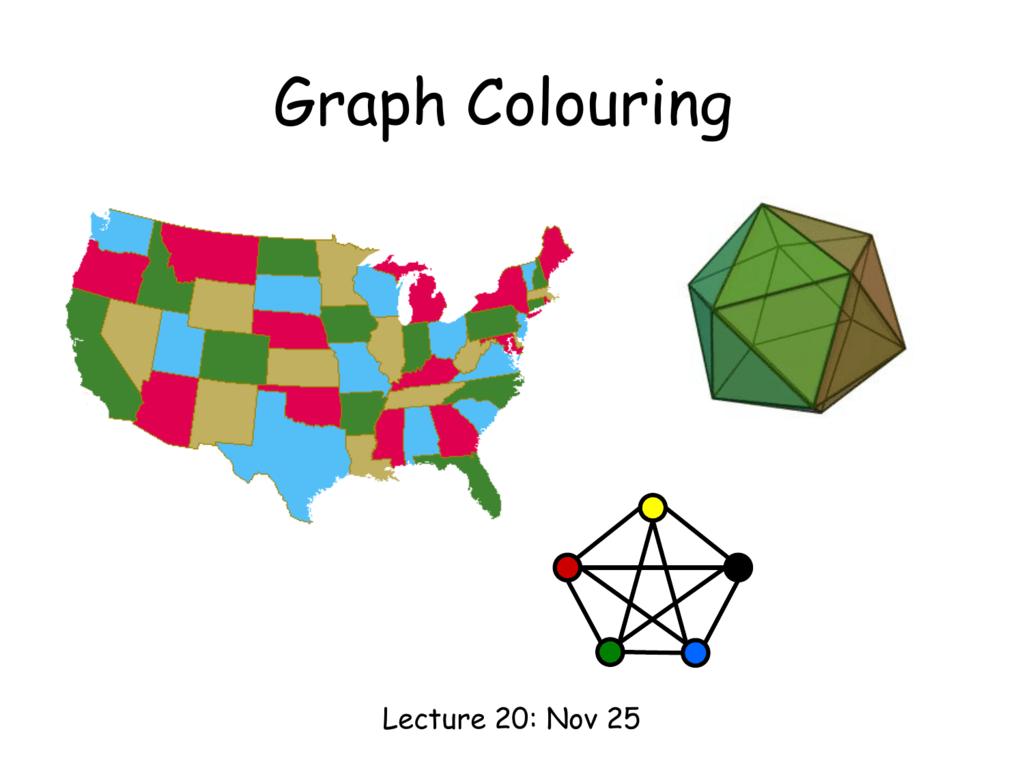
What is the maximum number of colors required to color the regions of a map.

Art edge coloring book. In its simplest form it is a way of coloring the vertices of a graph such that no two adjacent vertices are of the same color. Given a proper coloring of a graph g and a color class c such that none of its vertices have neighbors in all the. A k coloring of g is an assignment of k colors to the vertices of g in such a way that adjacent vertices are assigned different colors.

Under the umbrella of social networks are many different types of graphs. A graph coloring for a graph with 6 vertices. Graph coloring if you ever decide to create a map and need to color the parts of it optimally feel lucky because graph theory is by your side.

The graph coloring game is a mathematical game related to graph theory. Graph theory is the study of mathematical objects known as graphs which consist of vertices or nodes connected by edges. Acquaintanceship and friendship graphs describe whether people know each other.

Graph coloring is nothing but a simple way of labelling graph components such as vertices edges and regions under some constraints. It was first studied in the 1970s in independent papers by vizing and by erdos rubin and taylor. This question along with other similar ones have generated a lot of results in graph theory.

It is an assignment of labels traditionally called colors to elements of a graph subject to certain constraints. This function computes a b coloring with at most k colors that maximizes the number of colors if such a coloring exists. It is impossible to color the graph with 2 colors so the graph has chromatic number 3.

In a coloring game two players use a given set of colors to construct a coloring of a graph following specific rules depending on the game we considerone player tries to successfully complete the coloring of the graph when. This is called a vertex coloringsimilarly an edge coloring assigns a color to each. In graph theory a branch of mathematics list coloring is a type of graph coloring where each vertex can be restricted to a list of allowed colors.

Thus the graph is not planar. Let g be a graph with no loops. In graph theory graph coloring is a special case of graph labeling.

Graph theory is also widely used in sociology as a way for example to measure actors prestige or to explore rumor spreading notably through the use of social network analysis software. If g has a k coloring then g is said to be k coloring then g is said to be k colorablethe chromatic number of g denoted by xg is the smallest number k for which is k colorable. Any scenario in which one wishes to examine the structure of a network of connected objects is potentially a problem for graph theory.

A graph coloring is an assignment of labels called colors to the vertices of a graph such that no two adjacent vertices share the same color. In a graph no two adjacent vertices adjacent edges or adjacent regions are colored with minimum number of colors. Coloring game problems arose as game theoretic versions of well known graph coloring problems.