Coloring Of Graphs In Graph Theory Pdf, Decades Old Graph Problem Yields To Amateur Mathematician Quanta Magazine

So lets define that and then see prove some facts about it.

Food coloring is a solid. Perfect graphs are by definition colorable with the most limited palette possible. This is called a vertex coloringsimilarly an edge coloring assigns a color to each. In its simplest form it is a way of coloring the vertices of a graph such that no two adjacent vertices are of the same color.

Proof by induction on the number of vertices. When coloring a graph every node in a mutually connected cluster or clique must receive a distinct color so any graph needs at least as many colors as the number of nodes in its largest clique. Given a graph gve with n vertices and m edges the aim is to color the vertices of.

Routes between the cities can be represented using graphs. Graph theory 2 science. And almost you could almost say is a generic approach.

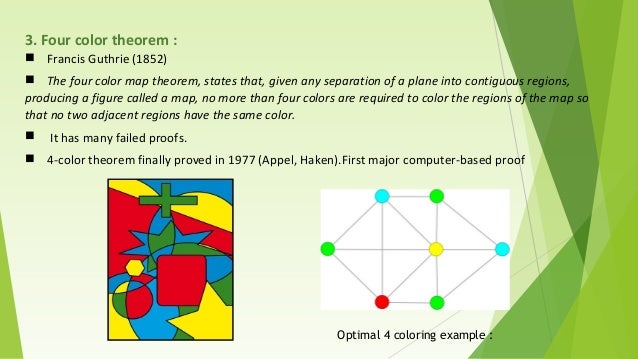
The parsing tree of a language and grammar of a language uses graphs. Any connected simple planar graph with 5 or fewer vertices is 5colorable. All connected simple planar graphs are 5 colorable.

While graph coloring the constraints that are set on the graph are colors order of coloring the way of assigning color etc. Most of the graph coloring algorithms in practice are based on this approach. In graph theory graph coloring is a special case of graph labeling.

A coloring is given to a vertex or a particular region. A star coloring of an undirected graph g is a proper vertex coloring of g ie no two neighbors are assigned the same color such that any path of length 3 in g is not bicolored. And that is probably the most basic graph coloring approach.

In this paper we give the exact value of the star chromatic. First let us define the constraint of coloring in a formal way coloring a coloring of a simple graph is the assignment of a color to each vertex of the graph such that no two adjacent vertices are assigned the same color. The star chromatic number of an undirected graph g denoted by x s g is the smallest integer k for which g admits a star coloring with k colors.

And for our graph g with. And were going to call it the basic graph coloring algorithm. Depicting hierarchical ordered information such as family tree can be used as a special.

This question along with other similar ones have generated a lot of results in graph theory. Graph coloring gcp is one of the most studied problems in both graph theory and combinatorial optimization. Proper coloring of a graph is an assignment of colors either to the vertices of the graphs.

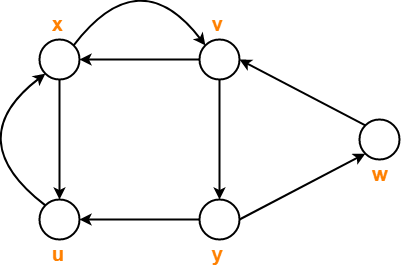
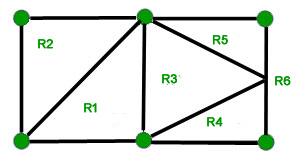
A graph g is a mathematical structure consisting of two sets vg vertices of g and eg edges of g. In most graphs you need many more colors than this.