Achromatic Coloring In Graph Theory, Pdf On Achromatic Coloring Of Star Graph Families Semantic Scholar

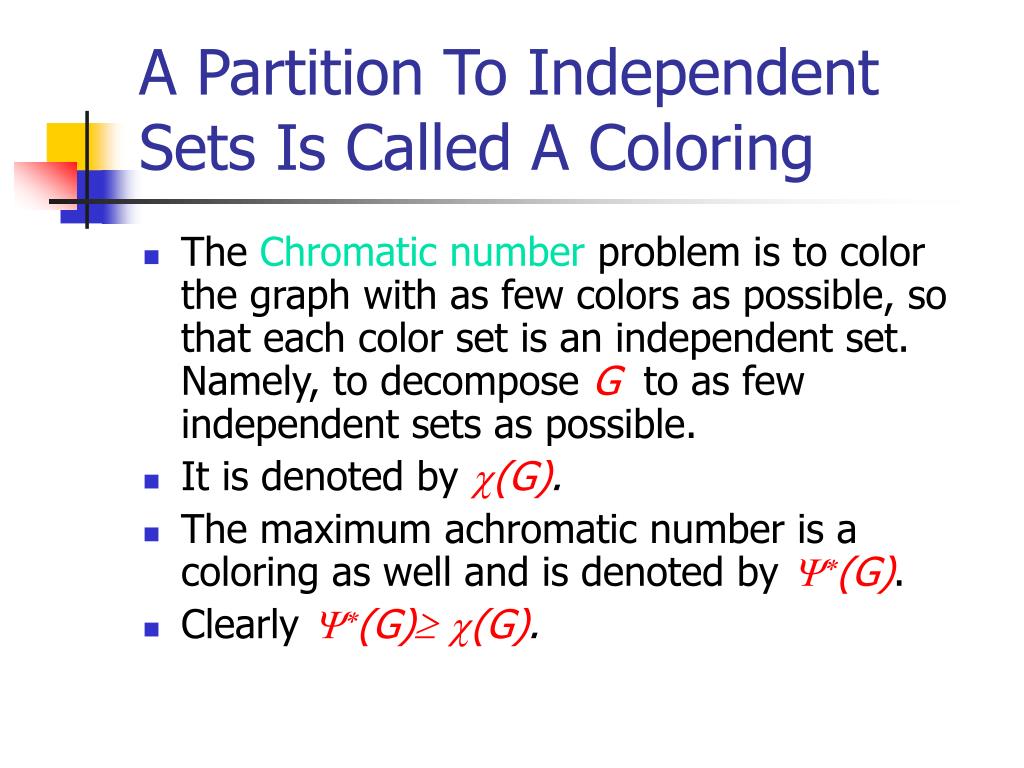
Clearly any graph g with p points has a p coloring but this coloring is complete only for the complete graph k.

Lego unicorn kitty coloring page. F this equation is what we are trying to solve here. Similarly a proper achromatic coloring of a graph gassigns colors to each vertex of gsuch that for each color class ci nci contains representativesof every color class. A graph coloring is an assignment of labels called colors to the vertices of a graph such that no two adjacent vertices share the same color.

2018 12 02 2018 06 26 by leo benkel. The largest possible number of colours in an achromatic colouring is called the achromatic number and is denoted by psletg be a finite undirected graph with no loops and multiple edges. On achromatic coloring of corona graphs let g vgeg be a simple graph and an achromatic coloring of g is a proper vertex coloring of g in which every pair of colors appears on at least one pair of adjacent vertices.

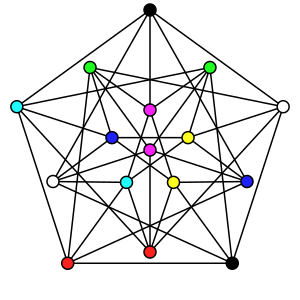
A graph coloring for a graph with 6 vertices. It is impossible to color the graph with 2 colors so the graph has chromatic number 3. At most one edge.

Graph coloring in graph theory graph coloring is a process of assigning colors to the vertices such that no two adjacent vertices get the same color. In graph theory complete coloring is the opposite of harmonious coloring in the sense that it is a vertex coloring in which every pair of colors appears on at least one pair of adjacent vertices. The maximum number of color classes in a proper achromatic partition of gis the.

Graph theory graph coloring and chromatic polynomial. Chromatic number of a graph is the minimum number of colors required to properly color the graph. Equivalently a complete coloring is minimal in the sense that it cannot be transformed into a proper coloring with fewer colors by merging pairs of color classes.

Number xg is the minimum number of colors required in any proper coloring of g. The achromatic number ps of a graph g is the maximum number of colors possible in any complete coloring of g. A proper vertex coloring of a graph g is achromatic respectively harmonious if every two colors appear together on at least one resp.

This article is a simple explanation on how to find the chromatic polynomial as well as calculating the number of color. The achromatic number hg of a graph g is the largest number m such that g has a complete m coloring.